Jet in a cross flow

Underlying Flow Regime 1-05 © copyright ERCOFTAC 2004

Description

Introduction

Relevance

Understanding the underlying flow regime (UFR) ‘jet in cross flow’ documented here is basic to solving several problems of considerable practical importance in aeronautics, civil engineering, environmental engineering and turbomachinery. This flow regime has been systematically studied since the first half of the twentieth century. In 1993 the Advisory Group for Aerospace Research and Development (AGARD) organized a conference with the title ‘Computational and Experimental Assessment of Jets in Cross Flow’. Thirty seven papers were presented, showing the vigour of this subject as a field of research.

Margason (1993) gives a list of the main applications of knowledge about jets in cross flow:

1) Plume dispersion from smoke stacks and volcanoes

This is a pollution problem where smoke concentration is of interest. The smoke leaves either the stack or the volcano with an upward momentum due to buoyancy into a stagnation air mass or into a cross wind. So it is usual to make the analysis of the motion caused by buyancy until it becomes negligible and then to consider a diffusion formulation.

2) Effluent dispersal into streams

This is also a pollution problem where the focus of research is diffusion of the jet into a lake or a river. The goal is the identification of the polluted region and the estimation of the concentration of pollutant. This analysis often happens in the design of sewage treatment and disposal systems.

3) Turbomachinery cooling and fuel injection

In cooling, the problem is to protect the components of the combustor or the turbine against very high temperatures, adopted for purposes of efficiency. The optimum design of a cooling system will protect the components using as little cooling medium as possible and affecting the efficiency of the component as little as possible. In fuel injection, the designer wishes to achieve the best possible quality of mix of fuel and air.

4) Control jets

On underwater vehicles and on aircraft, control jets are used to produce control moments. Interest here is on the interaction between different flows and their effect on the control moment.

5) Vertical and/or Short Take Off and Landing aircraft (V/STOL)

This has probably been the most important application of the study of jets in cross flow. The problem is to minimise the loss in lifting force and the nose-up pitching moment caused by the jet. Objectives also include the minimisation of ground damage, of modification of aerodynamic forces and moments and of ingestion of hot, exhausted gases in engine inlets.

The Physics of a Jet in Cross Flow

The physical phenomenon is described by several authors. However, some differences of interest imply different descriptions of the fundamental aspects of the flow regime. So, those who are interested in V/STOL, describe a system where we have a jet, a cross flow and a wall, normal to the jet exit. We will concentrate our attention on a flow system that is of interest to turbomachinery and environmental engineers.

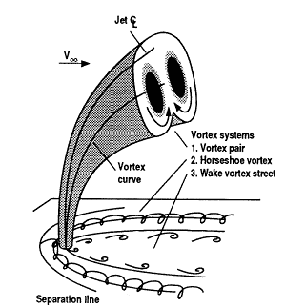
A jet exhausting into a cross flow follows a curved path downstream while its cross section changes (see Fig. 1.1). For the case of a circular jet (Margason (1993)), the pressure distribution due to potential flow around a rigid circular cylinder can be considered. The pressure coefficient is given by . At and there are stagnation points and C_p=1. At the lateral edges, where and the pressures reach a minimum and C_p=-3. The flow then spreads laterally into an oval shape. At the same time the cross flow shears the jet fluid along the lateral edges downstream to form a kidney shaped cross section. At increasing distances from the jet exit, the shearing folds the downstream face over itself to form a vortex pair. Fig. 1.1 also shows the secondary vortices: the horseshoe vortex and the wake vortex street.

Fig. 1.1 – The three vortex systems associated with the Jet in a Cross Flow Margason (1993)

The ratio R = (jet velocity / cross flow velocity) is of particular importance. When R is high (6, 8, 10 or more - see for example Keffer and Baines (1962)), the jet penetrates far across the main flow. If R is low, the jet tends to bow at a short distance from the orifice. Fig. 1.2 (a), from Andreopoulos and Rodi (1984) shows the case of R=0.5. The jet bends immediately after leaving the orifice. The main flow is slightly distorted by the jet and, downstream the orifice, it looks like a ‘cover’, under which the fluid of the jet flows. Fig. 1.2 (b) (same authors) shows the case of R=2. With a higher velocity, the jet penetrates into the cross stream before being bent over. The jet is weakly affected near the exit. In both cases, wakes with complex flow patterns are formed downstream the jet. Close to the wall, a region of reverse flow forms. Cross stream fluid that enters this region moves upstream, is lifted by the jet and then moves downstream with the fluid of the jet. As the jet is bent, its cross section is distorted by two counter rotating vortices, taking the shape of a kidney, as mentioned above. This secondary motion decays in the downstream direction under the action of turbulent stresses. The approaching boundary layer has negative vorticity, which is increased as the jet is bent. In the pipe, near the exit, the counter-rotating vorticity is formed by the coss stream moving around the jet.

The basic feature of Jet in Cross Flow is the mutual deflection of jet and cross flow. The jet is bent over by the cross stream, near the wall if R is low and far from the wall if R is high. The cross stream is deflected as if it had hit a rigid obstacle. The vortices form when the wall boundary layer encounters an adverse pressure gradient at the front of the jet and separates.

Fig. 1.2 – Flow development for R=0.5 (a) and for R=2.0 (b). Andreopoulos and Rodi (1984)

Review of UFR studies and choice of test case

Keffer and Baines (1962) carried out an experimental and analytical study of a jet directed normal to a uniform, steady cross-wind. They were concerned with the discharge of waste gases from chimney stacks. Velocity ratios of 2, 4, 6, 8 and 10 were considered. Velocity magnitude and direction were measured by hot wire techniques at a large number of points. Equations of continuity and momentum were written in natural coordinates, where the main axis was the centreline of the jet (maximum velocity). A numerical coefficient of the momentum equation was experimentally determined.

Crabb et al. (1981) used laser Doppler anemometry, hot wire anemometry and helium trace concentrations to study the mixing of jet and cross flow. They were mainly concerned with the problem of fuel and air mixing in combustors. They used velocity ratios of 1.15 and 2.3. They found that, in its mean flow characteristics, the jet in cross flow is controlled mainly by pressure forces and, in principle, the flow can be calculated without detailed knowledge of the structure of turbulence.

Andreopoulos and Rodi (1984) measured the three mean velocity components, the turbulent kinetic energy and the three turbulent shear stresses for velocity ratios 0.5, 1 and 2. This work will later be discussed in more detail.

Sherif and Pletcher (1989) measured velocity and turbulence characteristics of a round turbulent jet in cross flow. The experiments were conducted in a water channel and water was injected vertically upward from a pipe. Mean velocities, turbulence intensities, Reynolds stresses, structural parameters, correlation coefficients and turbulent kinetic energy were measured. Velocity ratios of 2, 4 and 6 were considered.

Kavsaoglu and Schetz (1989) conducted experimental work to study the effects of swirl and high turbulence in the exhaust of a circular jet injected at 90 degrees angle into a cross flow. Surface pressure distributions and mean velocity components were obtained for low exit turbulence, high exit turbulence and different swirl ratios (maximum peripheral velocity divided by the axial velocity). Velocity ratios of 2.2, 4 and 8 were considered.

As to analytical work, a pioneer work was that carried out by Keffer and Baines (1962), but more recent work is available, making use of powerful computational resources.

Bergeles et al. (1978) studied the turbulent jet in a cross stream at low injection rates. As they point out, this range of jet velocity / main stream velocity ratio – less than 1 – is the one to be considered by those researchers concerned with blade cooling, whilst higher ratios – higher than 1 – are of interest to those researchers concerned with smokestacks or VTOL for example. They extended the previous work of Pratap and Spalding (1974) to their analysis under the assumption that the main stream velocity in the main direction of the flow is an order of magnitude larger than those in planes normal to this direction and that re-circulation behind the jet can be neglected. The authors considered injection at 90 degrees and at 30 degrees. With 90 degrees the method proved good for velocity ratios up to 0.1 and with 30 degrees for velocity ratios up to 0.5. They used a turbulence model and a law of the wall. They were mainly concerned with blade cooling.

Yagci and Kavsaoglu (1993) carried out a Navier Stokes analysis of a subsonic swirling jet in subsonic cross flow, taking as a test case the experimental study made by Kavsaoglu and Schetz (1989). They used a three dimensional, thin layer, Reynolds averaged, compressible Navier Stokes code. Baldwin-Lomax turbulence model and the algebraic curved jet turbulence model of Oh and Schetz (1990) were used, depending on the location in the flow field. Characteristic boundary conditions based on Riemann invariants were utilized for the flow in and out planes. A single block grid with a cavity at the jet entrance was used.

Yuan et al. (1999) report large eddy simulations of a round jet normal to a cross flow, performed for R = 2.0 and R = 3.3 and Reynolds numbers 1050 and 2100, based on cross flow velocity and jet diameter. Calculated mean and turbulent statistics match experimental measurements reasonably well.

The experimental work by Andreopoulos and Rodi (1984) will be discussed here in more detail because of its high quality documentation and its completeness. It should always be considered as a reference for code validation. The analytical paper by Yagci and Kavsaoglu (1993) will be seen with the experimental work by Kavsaoglu and Schetz (1989) and the analytical work by Yuan et al. (1999) will be seen with the experimental work by Sherif and Pletcher (1989). These two analytical investigations differ substantially. One deals with compressible flow, the other with incompressible flow; one is based on more conventional models, requiring moderate computational power, the other is based on large eddy simulation, requiring massive computational power.

Contributors: Flavio Franco - ABB ALSTOM Power UK Ltd