Best Practice Advice AC6-05
Annular compressor cascade with tip clearance
Application Challenge 6-05 © copyright ERCOFTAC 2004
Best Practice Advice for the AC
Key Fluid Physics
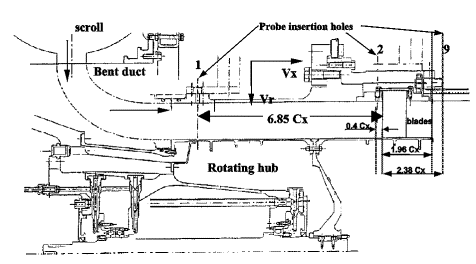
The “Annular Compressor Cascade with Tip-clearance” application challenge deals with the flow in a stationary cascade with tip-clearance. The cascade (see Figure 1) that features a hub-to-tip radius ratio of 0.75 has been designed by SNECMA for studying the tip-clearance effects in annular cascades at high but subsonic Mach numbers. The experiments (both pneumatic and LDV) have been performed for a mass flow rate of 13.2kg/s at the Laboratory of Thermal Turbomachinery of the National Technical University of Athens. The flow in the annular compressor cascade is of high-speed but subsonic and the Reynolds number is approximately 1.1∙106. The cascade consists of two sets of 19 untwisted blades, with constant, along the blade height, chord (C=100mm) that are placed at 51.4o stagger angle. They are used to form two alternative tip-clearances heights of 2% and 4%C, which are constant along the chord. In the stationary cascade of a rotor airfoil configuration adopted, the relative blade/end-wall motion was simulated by means of the inner wall rotating from pressure to suction side. In addition, experiments have been carried out for a still hub, to allow for the study of the effects that the relative end-wall/blade motion introduces to the flow. Four experimental cases arise from the combination of 2 tip-clearance heights and a rotating/still hub:
Figure 1: Layout of the testing section of the annular compressor cascade facility.
Case 1. The tip-clearance size is t/C=2% and the hub rotates at N=6540 rpm.
Case 2. The tip-clearance size is t/C=2% and the hub is still.
Case 3. The tip-clearance size is t/C=4% and a hub rotates at N=6540rpm.
Case 4. The tip-clearance size is t/C=4% and the hub is still.
As far the physics of the flow are of concern, the flow inside the cascade is characterised by the development of a complex vortical structure, whose relative strength and location as the flow moves downstream is mainly affected by the size of the tip-clearance and the rotation of the hub. The main vortex appearing is the tip-clearance one. In addition, an outer passage vortex close to the casing, which turns from the pressure to the suction side of the wall, and an inner passage vortex, which becomes displaced upwards by the leakage vortex, are two secondary vortices appearing that interact with other relevant underlying flow regimes as the boundary layers and the wakes of the blades.
The Underlying Flow Regime documentation available at the time of drafting of this document includes:
• UFR1-02: “Wing/Blade Tip and Tip-Clearance Flow,” version dated 3/2003.
• UFR2-04: “Flow Around Airfoils and Blades (Subsonic),” version dated 10/2003.
• UFR2-06: “Flow Around Airfoils and Blades (Transonic),” version dated 7/2003.
• UFR3-03: “2D Boundary Layer in an Adverse Pressure Gradient,” version dated 10/2003.
• UFR3-08: “3D Boundary Layers under Various Pressure Gradients, Including Severe Adverse Pressure Gradients Causing Separation,” Knowledge Base- html version.
• UFR3-10: “The Plane Wall Jet,” version 5/2003.
• UFR4-04: “Flow in a Curved Rectangular Duct,” version dated 6/2003
• UFR4-05: “Curved Duct/Passage Flow (Accelerating),” version 7/2003
On the other hand the following UFR documentation was not available and therefore not used:
• UFR2-05: “Flow Around Airfoils: A-airfoil”.
• UFR3-04: “Laminar-Turbulent Boundary Layer Transition”.
• UFR3-12: “Stagnation Point Flow”.
• UFR3-19: “By Pass Transition on a Flat Plate”.
• UFR4-01: ‘Secondary Flow in Rotating and Non-rotating Channels”.
• UFR2-07: “3D Flow around Blades”.
Application Uncertainties
Uncertainties for this application challenge are:
• No measurements of turbulent quantities have been reported.
• The only measurements upstream of the cascade available are at 40%C, which is relatively close to the blade for computational purposes (i.e. to impose the available measurements as inlet boundary conditions). A direct consequence of this is that there are no detailed distributions of flow quantities for inlet boundary conditions.
• In the data processing during the measurements, the flow was treated as isothermal (constant total temperature) even in the rotating hub cases. Therefore the fluid’s total energy increase in the, restricted, area near the rotating hub was not taken into account.
Computational Domain and Boundary Conditions
• The computational domain needs to extend downstream of the cascade by more than two chord lengths, to model both the rotating and the still part of the hub downstream of the cascade.
• Assess the effect of sensitivity to the inlet boundary conditions by varying the critical inputs with respect to the measured quantities at the 40%C upstream of the leading edge.
• According to UFR4-05 the radial equilibrium boundary condition must be employed at the outlet boundary. On the other hand, according to UFR1-02, a Neumann-type boundary condition should be imposed at the same plane. So, a Dirichlet-type boundary condition for the pressure at the outlet must be avoided.
Discretisation and Grid Resolution
• Use second order or higher discretisation schemes to reduce the numerical dissipation and to accurately compute the pressure-losses.
• Use a C- or an O-type grid around the airfoil to generate a regular structured grid in the leading edge region of a blade with a blunt leading edge.
• A solution adaptive grid is needed in the general case, with local refinement of the grid in areas of high gradients near the blade surfaces and in the tip-clearance and secondary flow vortices.
• In the absence of a solution adaptive grid:
o Use very fine grids (in both the stream-wise and the pitch-wise direction) to capture the boundary layer development on the suction side. Control the distance of the first node off the solid walls in conformity to the turbulence model in use.
o Use adequate grid resolution in the span-wise direction even outside the near-wall regions to accurately capture the vortices.
o The grid cell aspect ratio must be close to one in the vicinity of the vortex core.
o Perform grid dependency studies to eliminate grid sensitivity.
Physical Modelling
Turbulence Modelling for Qualitative Agreement:
• Correct behaviour of the flow quantities due to the tip-clearance size changes or the relative blade/end-wall motion, as well as the increase of losses in the presence of a still hub for the same value of the tip-clearance size are generally independent of the turbulence model in use.
• The flow shall be treated as isothermal even in the cases with a rotating hub, in conformity to the experimental data processing.
• Quantitative agreement of the flow quantities strongly depends on the turbulence model in use.
Turbulence Modelling:
• Standard k-ε models are unable to describe the turbulence in rotation-dominated flows satisfactorily and Reynolds stress models are needed for this. Standard k-ε models are less accurate in the prediction of the stream-wise velocity and static pressure in the core of vortices. They also over-predict the decay of the vortex.
• Use non-linear low-Reynolds-number models to capture the effect of wall anisotropy and flow curvature in adverse pressure gradients flow configuration.
• If a k-ε low-Reynolds-number model is to be used, the Chien model is preferable compared to the Yang-Shih one.
Near wall Model:
• The value of y+ at the first nodes off the solid boundaries must be in accordance with the general requirements of the turbulence model in use in order to give accurate results for the boundary layers on the solid surfaces.
• For the standard low-Reynolds-number k-ε model this value is 1.
• Since they are no measurements of the turbulent quantities to define the kind of transition type, no particular advice can be given for transition modelling. Nevertheless, a sensitivity study on the effect of transition is recommended.
Recommendations for Future Work
Further simulations of this application challenge are needed in order to come up with more up-dated and generally valid statements of advice.
The new simulations need to extend the validation of turbulence models that has already been carried out using this application challenge. It is recalled that computations for this application challenge incorporate the Baldwin-Lomax algebraic model, a mixing length model, the standard high-Reynolds-number k-ε model, the Launder-Sharma low-Reynolds-number k-ε model, the Yang-Shih linear k-ε model and the Craft-Launder-Sharma non-linear k-ε turbulent model.
The new simulations should contribute to the validation of modern turbulence models, as the k-ω SST and the V2F models by Menter and the model of Spalart-Allmaras among others. These models demonstrate good near-wall flow predictions and worth being investigated. In addition, Reynolds stress transport models (both differential and algebraic) that successfully predict the strong suppression of turbulence in rotation-dominated vortex core and a reasonable decay of the vortex should be also evaluated using this application challenge.
Finally sensitivity studies with respect to the inlet boundary conditions and the turbulence quantities should be carried out in order to remove the limitations of the measurements.
© copyright ERCOFTAC 2004
Contributors: Dr. E.S. Politis; Prof. K.D. Papailiou - NTUA
Site Design and Implementation: Atkins and UniS