Description AC2-12: Difference between revisions
m (Dave.Ellacott moved page Lib:Description AC2-12 to Description AC2-12 over redirect) |
|||
| (14 intermediate revisions by the same user not shown) | |||
| Line 8: | Line 8: | ||
=Description= | =Description= | ||
==Introduction== | ==Introduction== | ||
Turbulent separated bluff-body flows are encountered in many industrial applications, such as external aerodynamics and gas turbine combustors. This type of flow is associated with separation of the boundary layers, vortex shedding and bluff-body stabilized combustion and has long been of interest to scientists and engineers. The goal of the study on which this AC is based has been to replicate experiments carried out in a test rig at Volvo [1,2,3]. Due to the simple geometry, this test case is quite attractive for verifying and validating new algorithms and models in the frame of computational fluid dynamics (hereafter CFD). The knowledge obtained can be applied to assess the predictive capabilities of the state-of-the-art CFD codes to model and simulate unsteady combustion physics. The numerical results reported here are based on the work published in [4]. | Turbulent separated bluff-body flows are encountered in many industrial applications, such as external aerodynamics and gas turbine combustors. This type of flow is associated with separation of the boundary layers, vortex shedding and bluff-body stabilized combustion and has long been of interest to scientists and engineers. The goal of the study on which this AC is based has been to replicate experiments carried out in a test rig at Volvo [[Best_Practice_Advice_AC2-12#1|[1,2,3]]]. | ||
Due to the simple geometry, this test case is quite attractive for verifying and validating new algorithms and models in the frame of computational fluid dynamics (hereafter CFD). The knowledge obtained can be applied to assess the predictive capabilities of the state-of-the-art CFD codes to model and simulate unsteady combustion physics. The numerical results reported here are based on the work published in | |||
[[Best_Practice_Advice_AC2-12#4|[4]]]. | |||
==Relevance to Industrial Sector== | ==Relevance to Industrial Sector== | ||
There are at least two fields of application, where turbulent bluff-body flows play a significant role. The first one is external (or internal) aerodynamics. The second one is combustion applications, where flame stabilization is achieved using bluff-bodies. The latter is one of the most used approaches in a variety of propulsion and industrial combustion systems. It is employed for supplementary firing in industrial boilers and heat recovery steam generators, and is also used in ramjet and turbojet afterburner systems [5]. In addition, it is often used in fundamental studies (both experimental and numerical) of turbulent wakes and flame characteristics or as computational test case for the development of new models [5]. | There are at least two fields of application, where turbulent bluff-body flows play a significant role. The first one is external (or internal) aerodynamics. The second one is combustion applications, where flame stabilization is achieved using bluff-bodies. The latter is one of the most used approaches in a variety of propulsion and industrial combustion systems. It is employed for supplementary firing in industrial boilers and heat recovery steam generators, and is also used in ramjet and turbojet afterburner systems [[Best_Practice_Advice_AC2-12#5|[5]]]. | ||
In addition, it is often used in fundamental studies (both experimental and numerical) of turbulent wakes and flame characteristics or as computational test case for the development of new models [[Best_Practice_Advice_AC2-12#5|[5]]]. | |||
==Design or Assessment Parameters== | ==Design or Assessment Parameters== | ||
The flow dynamics of bluff-body flames can be assessed using both integral parameters and first and second order local statistics. The prime integral parameters are the recirculation zone length and the Strouhal number of convective and absolute instabilities, as well as first and second order statistics for the local velocity and scalar (temperature, species) distributions as well as their spectral density. Additionally, the combustion dynamics and lean blowoff (LBO) can be used to predict unsteady combustion physics. | The flow dynamics of bluff-body flames can be assessed using both integral parameters and first and second order local statistics. The prime integral parameters are the recirculation zone length and the Strouhal number of convective and absolute instabilities, as well as first and second order statistics for the local velocity and scalar (temperature, species) distributions as well as their spectral density. Additionally, the combustion dynamics and lean blowoff (LBO) can be used to predict unsteady combustion physics. | ||
==Flow Domain Geometry== | ==Flow Domain Geometry== | ||
Figure 1 shows a schematic drawing of the flow configuration. The set-up consisted of a straight channel with a rectangular cross-section, divided into an inlet section of 0.5 m length and a channel passage section of length L = 1 m and 0.12 m | [[Description_AC2-12#figure1|Figure 1]] | ||
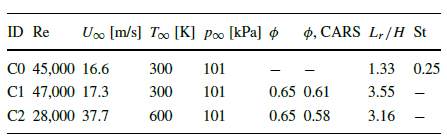
The principal flow parameters and experimental conditions are summarized in Table 1 for non-reactive and reactive cases (propane is the fuel), where the Reynolds number is based on the bluff-body side length, St is the Strouhal number, U is velocity, T is temperature, p is the static pressure, φ is the equivalence ratio and Lr/H represents the recirculation zone length. The symbol ∞ denotes that a parameter is applied at the rig inlet. The laminar flames speeds for the reacting cases C1 and C2 were set as Sl = 0.14 m/s and Sl = 0.77 m/s, respectively. | shows a schematic drawing of the flow configuration. The set-up consisted of a straight channel with a rectangular cross-section, divided into an inlet section of 0.5 m length and a channel passage section of length L = 1 m and 0.12 m × 0.24 m cross-section. The inlet section was used for flow straightening and turbulence control. The air entering the inlet section was distributed over the cross-section by a critical plate that, at the same time, isolated the channel acoustically from the air supply system. The channel passage section ended in a circular duct with a large diameter. The triangular bluff-body (with side length, H = 0.04 m) was mounted with its reference position 0.681 m upstream of the channel exit. | ||
The principal flow parameters and experimental conditions are summarized in [[Description_AC2-12#table1|Table 1]] | |||
for non-reactive and reactive cases (propane is the fuel), where the Reynolds number is based on the bluff-body side length, St is the Strouhal number, U is velocity, T is temperature, p is the static pressure, φ is the equivalence ratio and Lr/H represents the recirculation zone length. The symbol ∞ denotes that a parameter is applied at the rig inlet. The laminar flames speeds for the reacting cases C1 and C2 were set as Sl = 0.14 m/s and Sl = 0.77 m/s, respectively. | |||
<div id="figure1"></div> | <div id="figure1"></div> | ||
| Line 22: | Line 30: | ||
|align="center"|[[Image:AC2-12_fig1.png|650px]] | |align="center"|[[Image:AC2-12_fig1.png|650px]] | ||
|- | |- | ||
|align="center"|''' | |align="center"|'''Figure 1:''' The sketch of the Volvo rig. All linear dimensions are in mm | ||
|} | |} | ||
| Line 28: | Line 36: | ||
<div id="table1"></div> | <div id="table1"></div> | ||
{|align="center" border=0 | {|align="center" border=0 | ||
|align="center"|''' | |align="center"|'''Table 1:''' . Flow parameters used for the Volvo rig: inert (C0) and reactive (C1-C2) cases | ||
|- | |- | ||
|align="center"|[[Image:AC2-12_tab1.png|447px]] | |align="center"|[[Image:AC2-12_tab1.png|447px]] | ||
|} | |||
==Flow Physics and Fluid Dynamics Data== | |||
The Reynolds numbers based on the side length of the bluff-body and bulk velocity are estimated as, Re = 28,000 – 47,000 (sub-critical flow regime). The combustion is characterized by the lean, premixed propane-air mixture of equivalence ratio φ = 0.58 – 0.65 (“thin reaction zone” regime). The key features of the flow mechanics are the laminar boundary layer, separated shear layer, wake and the flow instabilities that provide complex, nonlinear interaction between them. The wake is dominated by two types of instabilities: convective instabilities or asymmetric vortex shedding (Bénard/von Kármán instability) and Kelvin–Helmholtz instability (sometimes called absolute) of the separated shear layer. For the reactive cases, the flame introduces additional phenomena through effects of exothermicity and flow dilatation on the flow field, which leads to the large differences between the non-reacting and the reacting wake. [[Description_AC2-12#figure2|Figure 2]] compares instantaneous and time-averaged flow visualizations of non-reactive and reactive wakes, obtained during the present numerical simulations. The key flow features are shown as well. | |||
<div id="figure2"></div> | |||
{|align="center" border=0 | |||
|- | |||
|align="center"|[[Image:AC2-12_fig2.png|650px]] | |||
|- | |||
|align="center"|'''Figure 2:''' The flow mechanics of the considered non-reactive and reactive bluff-body flows. The images are based on the results obtained by LES | |||
|} | |} | ||
<br/> | <br/> | ||
Latest revision as of 11:14, 16 August 2019
Turbulent separated inert and reactive flows over a triangular bluff body
Application Challenge AC2-12 © copyright ERCOFTAC 2019
Description
Introduction
Turbulent separated bluff-body flows are encountered in many industrial applications, such as external aerodynamics and gas turbine combustors. This type of flow is associated with separation of the boundary layers, vortex shedding and bluff-body stabilized combustion and has long been of interest to scientists and engineers. The goal of the study on which this AC is based has been to replicate experiments carried out in a test rig at Volvo [1,2,3]. Due to the simple geometry, this test case is quite attractive for verifying and validating new algorithms and models in the frame of computational fluid dynamics (hereafter CFD). The knowledge obtained can be applied to assess the predictive capabilities of the state-of-the-art CFD codes to model and simulate unsteady combustion physics. The numerical results reported here are based on the work published in [4].
Relevance to Industrial Sector
There are at least two fields of application, where turbulent bluff-body flows play a significant role. The first one is external (or internal) aerodynamics. The second one is combustion applications, where flame stabilization is achieved using bluff-bodies. The latter is one of the most used approaches in a variety of propulsion and industrial combustion systems. It is employed for supplementary firing in industrial boilers and heat recovery steam generators, and is also used in ramjet and turbojet afterburner systems [5]. In addition, it is often used in fundamental studies (both experimental and numerical) of turbulent wakes and flame characteristics or as computational test case for the development of new models [5].
Design or Assessment Parameters
The flow dynamics of bluff-body flames can be assessed using both integral parameters and first and second order local statistics. The prime integral parameters are the recirculation zone length and the Strouhal number of convective and absolute instabilities, as well as first and second order statistics for the local velocity and scalar (temperature, species) distributions as well as their spectral density. Additionally, the combustion dynamics and lean blowoff (LBO) can be used to predict unsteady combustion physics.
Flow Domain Geometry
Figure 1 shows a schematic drawing of the flow configuration. The set-up consisted of a straight channel with a rectangular cross-section, divided into an inlet section of 0.5 m length and a channel passage section of length L = 1 m and 0.12 m × 0.24 m cross-section. The inlet section was used for flow straightening and turbulence control. The air entering the inlet section was distributed over the cross-section by a critical plate that, at the same time, isolated the channel acoustically from the air supply system. The channel passage section ended in a circular duct with a large diameter. The triangular bluff-body (with side length, H = 0.04 m) was mounted with its reference position 0.681 m upstream of the channel exit.
The principal flow parameters and experimental conditions are summarized in Table 1 for non-reactive and reactive cases (propane is the fuel), where the Reynolds number is based on the bluff-body side length, St is the Strouhal number, U is velocity, T is temperature, p is the static pressure, φ is the equivalence ratio and Lr/H represents the recirculation zone length. The symbol ∞ denotes that a parameter is applied at the rig inlet. The laminar flames speeds for the reacting cases C1 and C2 were set as Sl = 0.14 m/s and Sl = 0.77 m/s, respectively.

|
| Figure 1: The sketch of the Volvo rig. All linear dimensions are in mm |
| Table 1: . Flow parameters used for the Volvo rig: inert (C0) and reactive (C1-C2) cases |
|
Flow Physics and Fluid Dynamics Data
The Reynolds numbers based on the side length of the bluff-body and bulk velocity are estimated as, Re = 28,000 – 47,000 (sub-critical flow regime). The combustion is characterized by the lean, premixed propane-air mixture of equivalence ratio φ = 0.58 – 0.65 (“thin reaction zone” regime). The key features of the flow mechanics are the laminar boundary layer, separated shear layer, wake and the flow instabilities that provide complex, nonlinear interaction between them. The wake is dominated by two types of instabilities: convective instabilities or asymmetric vortex shedding (Bénard/von Kármán instability) and Kelvin–Helmholtz instability (sometimes called absolute) of the separated shear layer. For the reactive cases, the flame introduces additional phenomena through effects of exothermicity and flow dilatation on the flow field, which leads to the large differences between the non-reacting and the reacting wake. Figure 2 compares instantaneous and time-averaged flow visualizations of non-reactive and reactive wakes, obtained during the present numerical simulations. The key flow features are shown as well.

|
| Figure 2: The flow mechanics of the considered non-reactive and reactive bluff-body flows. The images are based on the results obtained by LES |
Contributed by: D.A. Lysenko and M. Donskov — 3DMSimtek AS, Sandnes, Norway
© copyright ERCOFTAC 2019